Refraction
Refraction is the bending of light waves that happens when light travels at anything other than normal incidence between two materials with different optical densities. Refraction effects occur all the time around us and explain why items inside glass or under water appear in slightly different positions and also why cut diamonds glint and glitter more than other gems.
Interactive Example: Use this tool to experiment with different incidence angles and refractive indexes to see the impact they have on refraction effects.
Refraction is also the principle behind magnifying glasses, lenses and most other optical equipment. The bending of different wavelengths by different amounts is also why we can see rainbow colour effects when light passes through a triangular prism or even a water droplet.
Definition
The refractive index of a material is the value that governs refraction. It is the ratio between the speed of light in a vacuum compared to the apparent speed of light within that material. The basic relationship is as follows:
where:
- n is the refractive index of a material,
- Cv is the speed of light in a vacuum (2.99792458 × 108 m/s), and
- Cm is the apparent speed of light within that material (m/s),
Along with the angle of incidence, the refractive index is used to determine both the amount of light reflected and the angle of refraction of any light that is transmitted (see Units and Measures section below).
Materials with higher refractive index values are typically denser than those with lower values. Optical density is inversely related to the apparent speed with which light passes through a material. It is this change in the apparent speed of light that causes refraction.
Apparent Speed of Light
When light travels through a vacuum, it travels at a velocity of 2.99792458 × 108 m/s. This is a universal constant C that we call the ‘Speed of Light’ and, as Einstein outlined in his Special Theory of Relativity, represents the fastest speed anything can ever travel in our universe.
However, when light travels through any other material, be it a solid, liquid or gas, it appears to do so at slower speeds. The reason is that materials other than a vacuum contain many molecules and sub-atomic particles which the light can collide with and bounce off. If each photon of light is continually bumping into things, it ends up taking a much more circuitous route within the material instead of just travelling straight though. Generally, the higher the density of the material, the more things in there to hit - which means the more circuitous the route taken by each photon and the slower the apparent speed of light within it will be.
At a micro level, the speed that photons travel between each collision is still the same as it is in a vacuum, so it is still a universal constant. It’s just that the extra distance travelled by each photon through the material is not something we can easily measure. What we can measure is the time delay between light entering a particular sample of a material and the same light exiting. This is why it is termed the apparent speed of light, as it only appears to travel slower, but is still a very useful property that is used in many light and optics calculations.
It should also be noted that even opaque materials can have a refractive index, it is just that the light will not travel very far into the material before it is entirely absorbed, making it difficult to measure and of very limited consequence.
Units and Measures
The refractive index is a unit-less ratio. The refractive index of a vacuum is 1.0, which is the lowest possible value as nothing can theoretically travel faster than the speed of light in a vacuum. For all other materials it will be larger than 1.0. Air, for example, has a value of 1.000293, water is 1.333 and Diamond is 2.418. For a more detailed list, see the Example Values section below.
Angle of Refraction
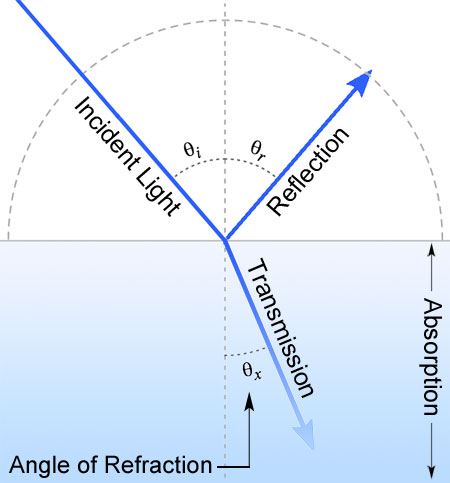
Figure 1: The angle of refraction when light passes into an optically dense transparent material.
If the refractive index of a material and the angle of incidence of a beam of light is known, then the angle of refraction is given by Snell's Law, which is as follows.
This can be simply re-arranged to give the angle of refraction:
where:
- n1 is the refractive index of the medium the light is travelling from,
- n2 is the refractive index of the medium the light is travelling into,
- θi is the angle of incidence, in degrees or radians, and
- θx is the angle of refraction, in degrees or radians.
The specification of degrees or radians means that it depends upon which maths library you are using and how it requires you to provide angle input for the sin and cos trigonometric functions. If you are using a pocket calculator, you can usually switch modes and enter either degrees or radians. If you are implementing these equations in computer code, you will very likely need to use radians.
Example Values
The following are some representative refractive indices for various materials, gasses and liquids that I have collected over the years. I used to have solid web references for most of them, but those links have long since moved on or died, so probably best to treat these values as indicative. A great reference to check for accurate values is http://refractiveindex.info/.
| GLASSES | RI |
|---|---|
| Glass (Average) | 1.5 |
| Glass, Fused Silica | 1.459 |
| Glass, Pyrex | 1.474 |
| Glass, Albite | 1.489 |
| Glass, Crown, Zinc | 1.517 |
| Glass, Crown | 1.52 |
| Glass, Flint, 29% lead | 1.569 |
| Glass, Flint, Light | 1.58 |
| Glass, Flint, Medium | 1.627 |
| Glass, Flint, Heavy | 1.655 |
| Glass, Flint, Dense | 1.66 |
| Glass, Flint, 55% lead | 1.669 |
| Glass, Flint, Lanthanum | 1.8 |
| Glass, Flint, 71% lead | 1.805 |
| Glass, Flint, Heaviest | 1.89 |
| Glass, Arsenic Trisulfide | 2.04 |
Table 1: Refractive indexes for different glasses.
| GASSES | RI |
|---|---|
| Vacuum | 1.000 |
| Argon | 1.000 |
| Carbon dioxide | 1.000 |
| Helium | 1.000036 |
| Hydrogen | 1.000132 |
| Air | 1.000293 |
| Chlorine gas | 1.001 |
Table 2: Refractive indexes for different gasses.
| LIQUIDS | RI |
|---|---|
| Liquid helium | 1.025 |
| Liquid Hydrogen | 1.097 |
| Liquid Carbon Dioxide | 1.2 |
| Liquid Nitrogen | 1.205 |
| Liquid Oxygen | 1.221 |
| Water (100 degC) | 1.318 |
| Methanol | 1.329 |
| Water (0 degC) | 1.333 |
| Silicone oil | 1.336 - 1.582 |
| 10% Sugar solution in water | 1.3477 |
| Acetone | 1.36 |
| Ethanol | 1.36 |
| Vodka | 1.363 |
| 25% Sugar solution in water | 1.3723 |
| Liquid Chlorine | 1.385 |
| Kerosene | 1.39 |
| 50% Sugar solution in water | 1.42 |
| Oil, vegetable (50 degC) | 1.47 |
| Turpentine | 1.472 |
| Glycerol | 1.4729 |
| 75% Sugar solution in water | 1.4774 |
| Benzene | 1.501 |
| Honey, 13% water content | 1.504 |
| Carbon disulfide | 1.628 |
| Bromine | 1.661 |
Table 3: Refractive indexes for different liquids.
| SOLIDS | RI |
|---|---|
| Ice Water | 1.31 |
| Cryolite | 1.338 |
| Opal | 1.45 |
| Pyrex glass | 1.47 |
| Crown glass (impure) | 1.485 - 1.755 |
| Obsidian | 1.489 |
| Acrylic glass | 1.490 - 1.492 |
| Plexiglas | 1.5 |
| Crown glass (pure) | 1.50 - 1.54 |
| Halite (rock salt) | 1.516 |
| Flint glass (impure) | 1.523 - 1.925 |
| Quartz | 1.544 |
| Emerald | 1.56 - 1.605 |
| Polycarbonate | 1.6 |
| Flint glass (pure) | 1.60 - 1.62 |
| Topaz | 1.607 |
| Ruby | 1.757 |
| Sapphire | 1.762 – 1.778 |
| Cubic zirconia | 2.15 - 2.18 |
| Zinc oxide | 2.4 |
| Diamond | 2.418 |
| Silicon | 3.48 - 3.42 |
| Gallium phosphide | 3.5 |
| Gallium arsenide | 3.927 |
| Germanium | 4.05 - 4.01 |
Table 4: Refractive indexes for different solids.
References
Speed of Light
Refractive Index:
- http://refractiveindex.info/
- https://en.wikipedia.org/wiki/List_of_refractive_indices
- http://www.britannica.com/EBchecked/topic/495677/refractive-index
- http://www.matter.org.uk/Schools/SchoolsGlossary/refractive_index.html
- http://www.fpharm.uniba.sk/fileadmin/user_upload/english/Fyzika/Refractive_index.pdf
- http://en.wikipedia.org/wiki/Refractive_index
- http://www.pixelandpoly.com/ior.html
Click here to comment on this page.